Vertical Dilation of Functions - eMathInstruction

Name: ___________________________________
Date: _________________
VERTICAL DILATION OF FUNCTIONS
ALGEBRA 2 WITH TRIGONOMETRY
We have now seen how to shift and reflect functions, specifically in the context of parabolas. In this lesson we
will see how to stretch or compress a function in the vertical direction. This is known as vertically dilating a
function. The first exercise will illustrate this concept with three related parabolas.
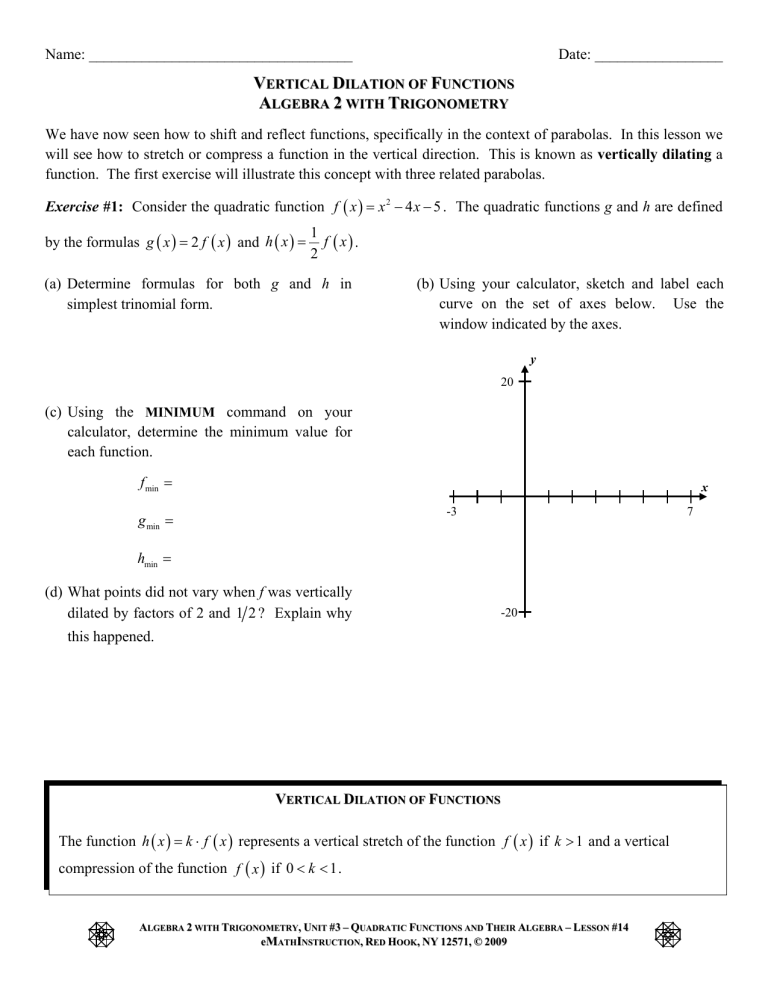
Exercise #1: Consider the quadratic function f ( x ) = x 2 в€’ 4 x в€’ 5 . The quadratic functions g and h are defined
by the formulas g ( x ) = 2 f ( x ) and h ( x ) =
1
f ( x) .
2
(a) Determine formulas for both g and h in
simplest trinomial form.
(b) Using your calculator, sketch and label each
curve on the set of axes below. Use the
window indicated by the axes.
y
20
(c) Using the MINIMUM command on your
calculator, determine the minimum value for
each function.
f min =
x
-3
g min =
7
hmin =
(d) What points did not vary when f was vertically
dilated by factors of 2 and 1 2 ? Explain why
-20
this happened.
VERTICAL DILATION OF FUNCTIONS
The function h ( x )= k в‹… f ( x ) represents a vertical stretch of the function f ( x ) if k > 1 and a vertical
compression of the function f ( x ) if 0 < k < 1 .
ALGEBRA 2 WITH TRIGONOMETRY, UNIT #3 – QUADRATIC FUNCTIONS AND THEIR ALGEBRA – LESSON #14
eMATHINSTRUCTION, RED HOOK, NY 12571, В© 2009
Exercise #2: If the point ( в€’3, 12 ) lies on the graph of the function y = f ( x ) , which of the following points
must lie on the graph of y = 3 f ( x ) ?
(1) ( в€’9, 36 )
(3) ( в€’3, 4 )
(2) ( в€’3, 36 )
(4) ( в€’9, 12 )
Exercise #3:
The graph of y = f ( x ) is shown below.
=
g ( x) 2 f ( x) в€’ 3.
Consider the function y = g ( x ) defined by
y
(a) What two transformations have occurred to the graph of
f in order to produce the graph of g? Specify both the
transformations and their order.
x
(b) Graph and label y = g ( x )
Exercise #4: The function h ( x ) has a domain given by the interval and a range given by the interval [ 2, 10 ] .
f ( x)
The function f ( x ) is defined by =
(1) [10, 22]
(3) [15, 27 ]
(2) [8, 12 ]
(4) [ 6, 32 ]
3
h ( x ) + 8 . Which of the following gives the range of f ( x ) ?
2
Exercise #5: If the quadratic function g ( x ) has a y-intercept of 12 . Which of the following is true about the
function =
h ( x ) 3g ( x ) в€’ 5 ?
(1) It has a y-intercept of -5.
(2) It has a y-intercept of 21.
(3) It has a y-intercept of -15.
(4) It has a y-intercept of 31.
ALGEBRA 2 WITH TRIGONOMETRY, UNIT #3 – QUADRATIC FUNCTIONS AND THEIR ALGEBRA – LESSON #14
eMATHINSTRUCTION, RED HOOK, NY 12571, В© 2009
Name: ___________________________________
Date: _________________
VERTICAL DILATION OF FUNCTIONS
ALGEBRA 2 WITH TRIGONOMETRY - HOMEWORK
SKILLS
1. If the point ( в€’6, 10 ) lies on the graph of y = f ( x ) then which of the following points must lie on the graph
of y =
1
f ( x) ?
2
(1) ( в€’3, 5 )
(3) ( в€’6, 5 )
(2) ( в€’3, 10 )
(4) ( в€’12, 20 )
2. If the function h ( x ) is defined as vertical stretch by a factor of 2 followed by a reflection in the x-axis of the
function f ( x ) then h ( x ) =
(1) 2 f ( в€’ x )
(2)
1
f ( x)
2
(3) в€’
1
f ( x)
2
(4) в€’2 f ( x )
3. If the graph of y = x 2 is compressed by a factor of 3 in the y-direction and then shifted 4 units down, the
resulting graph would have an equation of
y
(1)=
1 2
x в€’4
3
в€’3 x 2 в€’ 4
(2) y =
в€’4 x 2 в€’ 3
(3) y =
1
в€’ x2 + 4
(4) y =
3
=
y
4. The quadratic function f ( x ) has a turning point at ( в€’3, 6 ) . The quadratic
turning point of
(1) ( в€’2, 9 )
(3) ( в€’3, 7 )
(2) (1, 7 )
(4) ( в€’1, 9 )
2
f ( x ) + 3 would have a
3
5. The function g ( x ) is defined by g ( x ) =
в€’5 f ( x ) + 4 . What three transformation have occurred to the
graph of f to produce the graph of g? Specify both the transformations and their order.
ALGEBRA 2 WITH TRIGONOMETRY, UNIT #3 – QUADRATIC FUNCTIONS AND THEIR ALGEBRA – LESSON #14
eMATHINSTRUCTION, RED HOOK, NY 12571, В© 2009
1
в€’ h ( x) + 3 .
6. The graph of y = h ( x ) is shown below. The function f ( x ) is defined by f ( x ) =
2
y
(a) What three transformations have occurred to the
graph of h to produce the graph of f ? Specify the
transformations and the order they occurred in.
x
(b) Graph and label the function f ( x ) on the grid
below that contains h ( x ) .
y
7. A parabola is shown graphed to the right that is a
transformation of y = x 2 . The transformation includes
a vertical stretch and a vertical shift. What are the
stretch and shift? Based on your answer, write an
equation for this parabola.
x
REASONING
8. The function h ( x ) is defined by the equation =
h ( x ) 4 f ( x ) в€’ 12 .
Determine two different sets of
transformations that could produce the graph of h ( x ) from the graph of f ( x ) . For each, specify two
transformations and the order in which they occurred. As a hint, write h ( x ) in its factored form.
ALGEBRA 2 WITH TRIGONOMETRY, UNIT #3 – QUADRATIC FUNCTIONS AND THEIR ALGEBRA – LESSON #14
eMATHINSTRUCTION, RED HOOK, NY 12571, В© 2009