Homework 6 - Mechanical Engineering

Homework 6
ME 121: Engineering Mechanics II
D. H. Kelley
62 points
1. A 6-lb weight is suspended from a spring having a stiffness k = 3 lb/in. If the weight is given an
upward velocity of 20 ft/s when it is 2 inches above its equilibrium position, determine the equation
which describes the motion and the maximum upward displacement of the weight, measured from the
equilibrium position. Assume positive displacement is downward. (6 points)
2. A block of mass m is suspended from two springs having stiffness k1 and k2 . Determine the equivalent
stiffness of a single spring, and determine the period of oscillation, if the springs are
(a) parallel to each other (4 points), and
(b) in series (4 points).
3. The cart shown in Fig. 1 has mass m and is attached to two springs, each having stiffness k, unstretched
length l0 , and stretched length l when the cart is in its equilibrium position. If the cart is displaced a
distance x0 such that both springs remain in tension (x + 0 < l в€’ l0 ), determine the natural frequency
of oscillation. (8 points)
Figure 1: Problem 3.
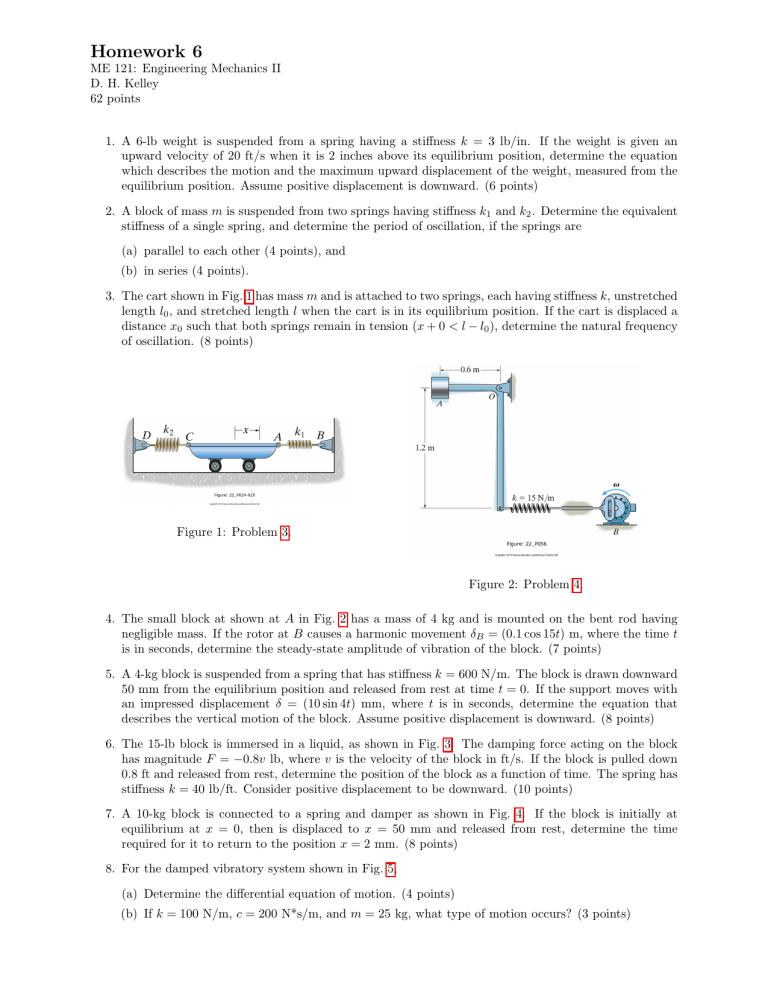
Figure 2: Problem 4.
4. The small block at shown at A in Fig. 2 has a mass of 4 kg and is mounted on the bent rod having
negligible mass. If the rotor at B causes a harmonic movement ОґB = (0.1 cos 15t) m, where the time t
is in seconds, determine the steady-state amplitude of vibration of the block. (7 points)
5. A 4-kg block is suspended from a spring that has stiffness k = 600 N/m. The block is drawn downward
50 mm from the equilibrium position and released from rest at time t = 0. If the support moves with
an impressed displacement Оґ = (10 sin 4t) mm, where t is in seconds, determine the equation that
describes the vertical motion of the block. Assume positive displacement is downward. (8 points)
6. The 15-lb block is immersed in a liquid, as shown in Fig. 3. The damping force acting on the block
has magnitude F = в€’0.8v lb, where v is the velocity of the block in ft/s. If the block is pulled down
0.8 ft and released from rest, determine the position of the block as a function of time. The spring has
stiffness k = 40 lb/ft. Consider positive displacement to be downward. (10 points)
7. A 10-kg block is connected to a spring and damper as shown in Fig. 4. If the block is initially at
equilibrium at x = 0, then is displaced to x = 50 mm and released from rest, determine the time
required for it to return to the position x = 2 mm. (8 points)
8. For the damped vibratory system shown in Fig. 5,
(a) Determine the differential equation of motion. (4 points)
(b) If k = 100 N/m, c = 200 N*s/m, and m = 25 kg, what type of motion occurs? (3 points)
Figure 4: Problem 7.
Figure 3: Problem 6.
Figure 5: Problem 8.
2