AP Physics Multiple Choice Practice – Kinematics Questions 1 – 3

AP Physics Multiple Choice Practice – Kinematics
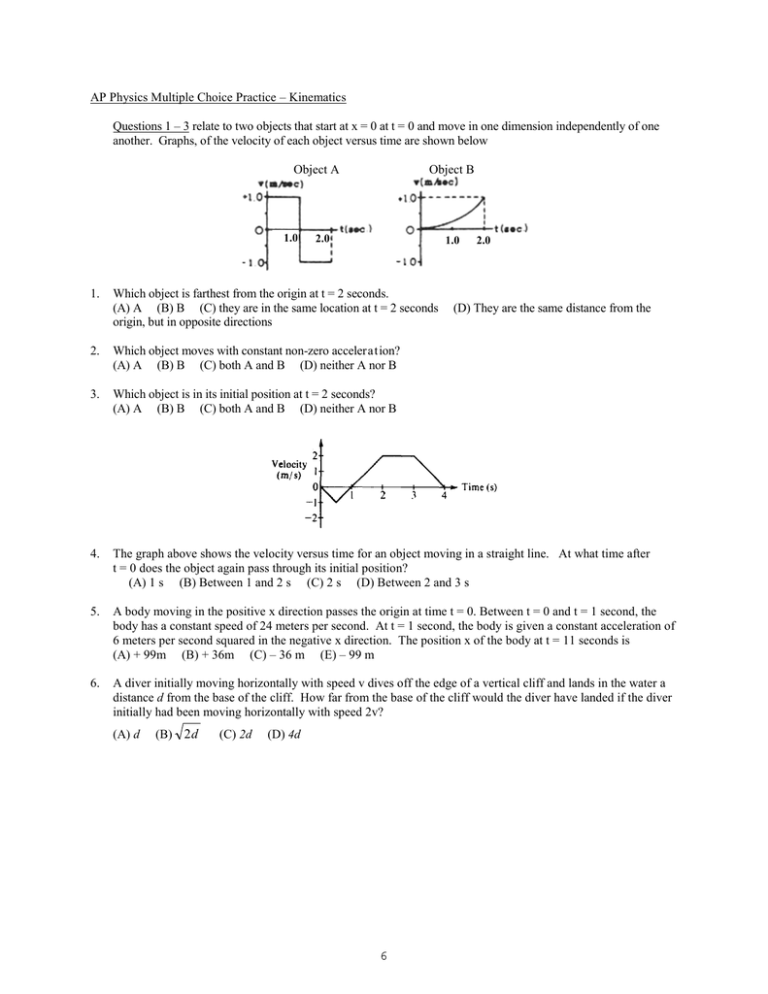
Questions 1 – 3 relate to two objects that start at x = 0 at t = 0 and move in one dimension independently of one
another. Graphs, of the velocity of each object versus time are shown below
Object A
1.0
Object B
2.0
1.0
2.0
1.
Which object is farthest from the origin at t = 2 seconds.
(A) A (B) B (C) they are in the same location at t = 2 seconds
origin, but in opposite directions
2.
Which object moves with constant non-zero acceleration?
(A) A (B) B (C) both A and B (D) neither A nor B
3.
Which object is in its initial position at t = 2 seconds?
(A) A (B) B (C) both A and B (D) neither A nor B
4.
The graph above shows the velocity versus time for an object moving in a straight line. At what time after
t = 0 does the object again pass through its initial position?
(A) 1 s (B) Between 1 and 2 s (C) 2 s (D) Between 2 and 3 s
5.
A body moving in the positive x direction passes the origin at time t = 0. Between t = 0 and t = 1 second, the
body has a constant speed of 24 meters per second. At t = 1 second, the body is given a constant acceleration of
6 meters per second squared in the negative x direction. The position x of the body at t = 11 seconds is
(A) + 99m (B) + 36m (C) – 36 m (E) – 99 m
6.
A diver initially moving horizontally with speed v dives off the edge of a vertical cliff and lands in the water a
distance d from the base of the cliff. How far from the base of the cliff would the diver have landed if the diver
initially had been moving horizontally with speed 2v?
(A) d
(B) 2 d
(C) 2d
(D) 4d
6
(D) They are the same distance from the
7.
A projectile is fired with initial velocity vo at an angle пЃ±0 with the horizontal and follows the trajectory shown
above. Which of the following pairs of graphs best represents the vertical components of the velocity and
acceleration, v and a, respectively, of the projectile as functions of time t?
Questions 8-9
A ball is thrown and follows the parabolic path shown above. Air friction is negligible.
point on the path. Points P and R are the same height above the ground.
Point Q is the highest
8.
How do the speeds of the ball at the three points compare?
(A) vP < vQ < vR (B) vR < vQ < vP (C) vQ < vR < vP (D) vQ< vP = vR
9.
Which of the following diagrams best shows the direction of the acceleration of the ball at point P?
(A)
(B)
(C)
(D)
10. The graph above represents position x versus time t for an object being acted on by a constant force. The
average speed during the interval between 1 s and 2 s is most nearly
(A) 2 m/s (B) 4 m/s (C) 5 m/s (D) 6 m/s
7
Questions 11 – 12
At time t = 0, car X traveling with speed v0 passes car Y which is just starting to move. Both cars then travel on
two parallel lanes of the same straight road. The graphs of speed v versus time t for both cars are shown above.
11. Which of the following is true at time t = 20 seconds?
(A) Car Y is behind car X. (B) Car Y is passing car X.
(D) Car X is accelerating faster than car Y.
(C) Car Y is in front of car X.
12. From time t = 0 to time t = 40 seconds, the areas under both curves are equal. Therefore, which of the
following is true at time t = 40 seconds?
(A) Car Y is behind car X. (B) Car Y is passing car X. (C) Car Y is in front of car X.
(d) Car X is accelerating faster than car Y.
13. Which of the following pairs of graphs shows the distance traveled versus time and the speed versus time for an
object uniformly accelerated from rest?
Speed
Distance
(A)
t
o
t
o
Speed
Distance
(C)
t
o
o
t
o
t
o
Speed
Distance
(E)
(D)
o
Speed
Distance
(B)
t
o
t
14. Vectors V1 and V2 shown above have equal magnitudes. The vectors represent the velocities of an object at
times t1, and t2, respectively. The average acceleration of the object between time t1 and t2 was
(A) directed north (B) directed west (C) directed north of east (D) directed north of west
8
t
AP Physics Free Response Practice – Kinematics
1982B1. The first meters of a 100-meter dash are covered in 2 seconds by a sprinter who starts from rest and
accelerates with a constant acceleration. The remaining 90 meters are run with the same velocity the sprinter
had after 2 seconds.
a. Determine the sprinter's constant acceleration during the first 2 seconds.
b. Determine the sprinters velocity after 2 seconds have elapsed.
c. Determine the total time needed to run the full 100 meters.
d. On the axes provided below, draw the displacement vs time curve for the sprinter.
2006B2. A world-class runner can complete a 100 m dash in about 10 s. Past studies have shown that runners in
such a race accelerate uniformly for a time t and then run at constant speed for the remainder of the race. A
world-class runner is visiting your physics class. You are to develop a procedure that will allow you to
determine the uniform acceleration a and an approximate value of t for the runner in a 100 m dash. By necessity
your experiment will be done on a straight track and include your whole class of eleven students.
(a) By checking the line next to each appropriate item in the list below, select the equipment, other than the runner
and the track, that your class will need to do the experiment.
____Stopwatches
____Metersticks
____Tape measures
____ Starter's pistol
____ Rulers
____ String
____ Masking tape
____ Chalk
(b) Outline the procedure that you would use to determine a and t, including a labeled diagram of the experimental
setup. Use symbols to identify carefully what measurements you would make and include in your procedure
how you would use each piece of the equipment you checked in part (a).
(c) Outline the process of data analysis, including how you will identify the portion of the race that has uniform
acceleration, and how you would calculate the uniform acceleration.
21
1993B1 (modified) A student stands in an elevator and records his acceleration as a function of time. The data are
shown in the graph above. At time t = 0, the elevator is at displacement x = 0 with velocity v = 0. Assume that
the positive directions for displacement, velocity, and acceleration are upward.
a. Determine the velocity v of the elevator at the end of each 5-second interval.
i. Indicate your results by completing the following table.
Time Interval (s)
0–5
5–10
10–15
15–20
v (m/s)
_____
_____
_____
_____
ii. Plot the velocity as a function of time on the following graph.
b.
Determine the displacement x of the elevator above the starting point at the end of each 5-second interval.
i. Indicate your results by completing the following table.
Time Interval (s)
0–5
5–10
10–15
15–20
x (m)
_____
_____
_____
_____
ii. Plot the displacement as a function of time on the following graph.
22
1994B1 (modified) A ball of mass 0.5 kilogram, initially at rest, is kicked directly toward a fence from a point 32
meters away, as shown above. The velocity of the ball as it leaves the kicker's foot is 20 meters per second at
an angle of 37В° above the horizontal. The top of the fence is 2.5 meters high. The ball hits nothing while in
flight and air resistance is negligible.
a. Determine the time it takes for the ball to reach the plane of the fence.
b. Will the ball hit the fence? If so, how far below the top of the fence will it hit? If not, how far above the top of
the fence will it pass?
c. On the axes below, sketch the horizontal and vertical components of the velocity of the ball as functions of time
until the ball reaches the plane of the fence.
23
AP Physics Multiple Choice Practice – Dynamics
SECTION A – Linear Dynamics
1.
A ball of mass m is suspended from two strings of unequal length as shown above. The magnitudes of the
tensions T1 and T2 in the strings must satisfy which of the following relations?
(A) Tl = T2 (B) T1 > T2
(C) T1 < T2
(D) Tl + T2 = mg
Questions 2 – 3
A 2-kg block slides down a 30В° incline as shown above with an acceleration of 2 m/s2.
2.
Which of the following diagrams best represents the gravitational force W. the frictional force f, and the normal
force N that act on the block?
(D)
3.
Which of the following correctly indicates the magnitudes of the forces acting up and down the incline?
(A) 20 N down the plane, 16 N up the plane
(B) 4 N down the plane, 4 N up the plane
(C) 0 N down the plane, 4 N up the plane
(D) 10 N down the plane, 6 N up the plane
4.
When the frictionless system shown above is accelerated by an applied force of magnitude the tension in the
string between the blocks is
(A) F (B) 2/3 F (C) ВЅ F (D) 1/3 F
5.
A ball falls straight down through the air under the influence of gravity. There is a retarding force F on the ball
with magnitude given by F = bv, where v is the speed of the ball and b is a positive constant. The ball reaches a
terminal velocity after a time t. The magnitude of the acceleration at time t/2 is
(A) Increasing
(B) Decreasing
(C) 10 m/s/s
(D) Zero
39
6.
A block of weight W is pulled along a horizontal surface at constant speed v by a force F, which acts at an angle
of пЃ± with the horizontal, as shown above. The normal force exerted on the block by the surface has magnitude
(A) greater than W
(B) greater than zero but less than W
(C) equal to W
(D) zero
7.
A uniform rope of weight 50 N hangs from a hook as shown above. A box of weight 100 N hangs from the
rope. What is the tension in the rope?
(A) 75 N throughout the rope
(B) 100 N throughout the rope
(C) 150 N throughout the rope
(D) It varies from 100 N at the bottom of the rope to 150 N at the top.
8.
When an object of weight W is suspended from the center of a massless string as shown above, the tension at
any point in the string is
(A) 2WcosпЃ±
(B) ВЅWcosпЃ±
(D) W/(2cosпЃ±)
(E) W/(cosпЃ±)
9.
A block of mass 3m can move without friction on a horizontal table. This block is attached to another block of
mass m by a cord that passes over a frictionless pulley, as shown above. If the masses of the cord and the pulley
are negligible, what is the magnitude of the acceleration of the descending block?
(A) g/4
(B) g/3
(C) 2g/3
(D) g
40
A plane 5 meters in length is inclined at an angle of 37В°, as shown above. A block of weight 20 N is placed at
the top of the plane and allowed to slide down.
10. The magnitude of the normal force exerted on the block by the plane is
(A) greater than 20 N
(B) greater than zero but less than 20 N
(C) equal to 20 N
(D) zero
11. Multiple correct: Three forces act on an object. If the object is moving to the right in translational equilibrium,
which of the following must be true? Select two answers.
(A) The vector sum of the three forces must equal zero.
(B) All three forces must be parallel.
(C) The magnitudes of the three forces must be equal.
(D) The object must be moving at a constant speed.
12. For which of the following motions of an object must the acceleration always be zero?
(A) Any motion in a straight line
(B) Simple harmonic motion
(C) Any motion at constant speed
(D) Any single object in motion with constant momentum
13. A rope of negligible mass supports a block that weighs 30 N, as shown above. The breaking strength of the
rope is 50 N. The largest acceleration that can be given to the block by pulling up on it with the rope without
breaking the rope is most nearly
(A) 6.7 m/s2
(B) 10 m/s2
(C) 16.7 m/s2
(D) 26.7 m/s2
Questions 14-15
A horizontal, uniform board of weight 125 N and length 4 m is supported by vertical chains at each end. A
person weighing 500 N is hanging from the board. The tension in the right chain is 250 N.
14. What is the tension in the left chain?
(A) 125 N
(B) 250 N
(C) 375 N
(D) 625 N
41
SECTION B – Circular Motion
1.
Multiple Correct: A person stands on a merry-go-round which is rotating at constant angular speed. Which of
the following are true about the frictional force exerted on the person by the merry-go-round? Select two
answers.
(A) The force is greater in magnitude than the frictional force exerted on the person by the merry-go-round.
(B) The force is opposite in direction to the frictional force exerted on the merry-go-round by the person.
(C) The force is directed away from the center of the merry-go-round.
(D) The force is dependent on the person's mass.
2.
A ball attached to a string is whirled around in a horizontal circle having a radius R. If the radius of the circle is
changed to 4R and the same centripetal force is applied by the string, the new speed of the ball is which of the
following?
(A) One-quarter the original speed
(B) One-half the original speed
(C) The same as the original speed
(D) Twice the original speed
3.
A racing car is moving around the circular track of radius 300 meters shown above. At the instant when the car's
velocity is directed due east, its acceleration is directed due south and has a magnitude of 3 meters per second
squared. When viewed from above, the car is moving
(A) clockwise at 30 m/s
(B) clockwise at 10 m/s
(C) counterclockwise at 30 m/s
(D) counterclockwise at 10 m/s
4.
The horizontal turntable shown above rotates at a constant rate. As viewed from above, a coin on the turntable
moves counterclockwise in a circle as shown. Which of the following vectors best represents the direction of the
frictional force exerted on the coin by the turntable when the coin is in the position shown?
(A)
5.
(B)
(C)
(D)
In which of the following situations would an object be accelerated? Select two answers.
(A) It moves in a straight line at constant speed.
(B) It moves with uniform circular motion.
(C) It travels as a projectile in a gravitational field with negligible air resistance.
(D) It is at rest.
50
6.
An automobile moves at constant speed down one hill and up another hill along the smoothly curved surface
shown above. Which of the following diagrams best represents the directions of the velocity and the
acceleration of the automobile at the instant that it is at the lowest position. as shown?
v
v
(A)
a
(B)
a
a
(C)
v
(D)
v
a
7.
A car initially travels north and then turns to the left along a circular curve. This causes a package on the seat of
the car to slide toward the right side of the car. Which of the following is true of the net force on the package
while it is sliding?
(A) The force is directed away from the center of the circle.
(B) There is not enough force directed north to keep the package from sliding.
(C) There is not enough force tangential to the car's path to keep the package from sliding.
(D) There is not enough force directed toward the center of the circle to keep the package from sliding.
8.
A child has a toy tied to the end of a string and whirls the toy at constant speed in a horizontal circular path of
radius R. The toy completes each revolution of its motion in a time period T. What is the magnitude of the
acceleration of the toy?
(A) Zero
9.
(B)
4пЃ° 2 R
T
2
(C)
пЃ°R
T2
(D) g
A compressed spring mounted on a disk can project a small ball. When the disk is not rotating, as shown in the
top view above, the ball moves radially outward. The disk then rotates in a counterclockwise direction as seen
from above, and the ball is projected outward at the instant the disk is in the position shown above. Which of
the following best shows the subsequent path of the ball relative to the ground?
51
10. A steel ball supported by a stick rotates in a circle of radius r, as shown above. The direction of the net force
acting on the ball when it is in the position shown is indicated by which of the following?
11. Inside a washing machine, the radius of the cylinder where the clothes sit is 0.50 m. In one of its settings the
machine spins the cylinder at 2.0 revolutions per second. What is the acceleration of an item of clothing?
(A) 0.080 m/s2 (B) 1.6 m/s2 (C) 8.0 m/s2 (D) 79 m/s2
12. A ball of mass m is attached to the end of a string of length Q as shown above. The ball is released from rest
from position P. where the string is horizontal. It swings through position Q. where the string is vertical, and
then to position R. where the string is again horizontal. What are the directions of the acceleration vectors of the
ball at positions Q and R?
Position Q
Position R
(A) Downward
Downward
(B) Downward
To the right
(C) Upward
Downward
(D) Upward
To the left
13. A mass m moves on a curved path from point X to point Y. Which of the following diagrams indicates a
possible combination of the net force F on the mass, and the velocity v and acceleration a of the mass at the
location shown?
52
AP Physics Free Response Practice – Dynamics
SECTION A – Linear Dynamics
1976B1. The two guide rails for the elevator shown above each exert a constant friction force of 100 newtons on the
elevator car when the elevator car is moving upward with an acceleration of 2 meters per second squared. The
pulley has negligible friction and mass. Assume g = 10 m/sec 2.
a.
On the diagram below, draw and label all forces acting on the elevator car. Identify the source of each force.
b.
Calculate the tension in the cable lifting the 400-kilogram elevator car during an upward acceleration of 2
m/sec2. (Assume g 10 m/sec2.)
Calculate the mass M the counterweight must have to raise the elevator car with an acceleration of 2 m/sec 2.
c.
1979B2. A 10-kilogram block rests initially on a table as shown in cases I and II above. The coefficient of sliding
friction between the block and the table is 0.2. The block is connected to a cord of negligible mass, which
hangs over a massless frictionless pulley. In case I a force of 50 newtons is applied to the cord. In case II an
object of mass 5 kilograms is hung on the bottom of the cord. Use g = 10 meters per second squared.
a. Calculate the acceleration of the 10-kilogram block in case I.
b. On the diagrams below, draw and label all the forces acting on each block in case II
10 kg
5 kg
c.
Calculate the acceleration of the 10-kilogram block in case II.
56
1982B2. A crane is used to hoist a load of mass m1 = 500 kilograms. The load is suspended by a cable from a hook
of mass m2 = 50 kilograms, as shown in the diagram above. The load is lifted upward at a constant acceleration
of 2 m/s2.
a. On the diagrams below draw and label the forces acting on the hook and the forces acting on the load as they
accelerate upward
b.
Determine the tension T 1 in the lower cable and the tension T 2 in the upper cable as the hook and load are
accelerated upward at 2 m/s2. Use g = 10 m/sВІ.
1985B2 (modified) Two 10-kilogram boxes are connected by a massless string that passes over a massless
frictionless pulley as shown above. The boxes remain at rest, with the one on the right hanging vertically and
the one on the left 2.0 meters from the bottom of an inclined plane that makes an angle of 60В° with the
horizontal. The coefficients of kinetic friction and static friction between the left-hand box and the plane are
0.15 and 0.30, respectively. You may use g = 10 m/s 2, sin 60В° = 0.87, and cos 60В° = 0.50.
a. What is the tension T in the string?
b. On the diagram below, draw and label all the forces acting on the box that is on the plane.
c.
Determine the magnitude of the frictional force acting on the box on the plane.
57
1986B1. Three blocks of masses 1.0, 2.0, and 4.0 kilograms are connected by massless strings, one of which passes
over a frictionless pulley of negligible mass, as shown above. Calculate each of the following.
a. The acceleration of the 4-kilogram block
b. The tension in the string supporting the 4-kilogram block
c. The tension in the string connected to the l-kilogram block
1987B1. In the system shown above, the block of mass M 1 is on a rough horizontal table. The string that attaches it
to the block of mass M2 passes over a frictionless pulley of negligible mass. The coefficient of kinetic friction
пЃ­k between M1 and the table is less than the coefficient of static friction пЃ­s
a. On the diagram below, draw and identify all the forces acting on the block of mass M1.
M1
b.
In terms of M1 and M2 determine the minimum value of пЃ­s that will prevent the blocks from moving.
c.
d.
The blocks are set in motion by giving M 2 a momentary downward push. In terms of M1, M2, пЃ­k, and g,
determine each of the following:
The magnitude of the acceleration of M 1
The tension in the string.
58
SECTION B – Circular Motion
1977 B2. A box of mass M, held in place by friction, rides on the flatbed of a truck which is traveling with constant
speed v. The truck is on an unbanked circular roadway having radius of curvature R.
a. On the diagram provided above, indicate and clearly label all the force vectors acting on the box.
b. Find what condition must be satisfied by the coefficient of static friction пЃ­ between the box and the truck bed.
Express your answer in terms of v, R, and g.
If the roadway is properly banked, the box will still remain in place on the truck for the same speed v even
when the truck bed is frictionless.
c.
d.
On the diagram above indicate and clearly label the two forces acting on the box under these conditions
Which, if either, of the two forces acting on the box is greater in magnitude?
1984B1. A ball of mass M attached to a string of length L moves in a circle in a vertical plane as shown above. At
the top of the circular path, the tension in the string is twice the weight of the ball. At the bottom, the ball just
clears the ground. Air resistance is negligible. Express all answers in terms of M, L, and g.
a. Determine the magnitude and direction of the net force on the ball when it is at the top.
b. Determine the speed vo of the ball at the top.
c.
d.
The string is then cut when the ball is at the top.
Determine the time it takes the ball to reach the ground.
Determine the horizontal distance the ball travels before hitting the ground.
73
1989B1. An object of mass M on a string is whirled with increasing speed in a horizontal circle, as shown above.
When the string breaks, the object has speed vo and the circular path has radius R and is a height h above the
ground. Neglect air friction.
a. Determine the following, expressing all answers in terms of h, vo, and g.
i. The time required for the object to hit the ground after the string breaks
ii. The horizontal distance the object travels from the time the string breaks until it hits the ground
iii. The speed of the object just before it hits the ground
b. On the figure below, draw and label all the forces acting on the object when it is in the position shown in the
diagram above.
.
c.
Determine the tension in the string just before the string breaks. Express your answer in terms of M, R, v o, & g.
74
1997B2 (modified) To study circular motion, two students use the hand-held device shown above, which consists of
a rod on which a spring scale is attached. A polished glass tube attached at the top serves as a guide for a light
cord attached the spring scale. A ball of mass 0.200 kg is attached to the other end of the cord. One student
swings the teal around at constant speed in a horizontal circle with a radius of 0.500 m. Assume friction and air
resistance are negligible.
a. Explain how the students, by using a timer and the information given above, can determine the speed of the ball
as it is revolving.
b. The speed of the ball is determined to be 3.7 m/s. Assuming that the cord is horizontal as it swings, calculate
the expected tension in the cord.
c. The actual tension in the cord as measured by the spring scale is 5.8 N. What is the percent difference between
this measured value of the tension and the value calculated in part b.?
d.
The students find that, despite their best efforts, they cannot swing the ball so that the cord remains exactly
horizontal.
i. On the picture of the ball below, draw vectors to represent the forces acting on the ball and identify the force
that each vector represents.
ii. Explain why it is not possible for the ball to swing so that the cord remains exactly horizontal.
iii. Calculate the angle that the cord makes with the horizontal.
1999B5 A coin C of mass 0.0050 kg is placed on a horizontal disk at a distance of 0.14 m from the center, as shown
above. The disk rotates at a constant rate in a counterclockwise direction as seen from above. The coin does not
slip, and the time it takes for the coin to make a complete revolution is 1.5 s.
a. The figure below shows the disk and coin as viewed from above. Draw and label vectors on the figure below to
show the instantaneous acceleration and linear velocity vectors for the coin when it is at the position shown.
b.
c.
d.
Determine the linear speed of the coin.
The rate of rotation of the disk is gradually increased. The coefficient of static friction between the coin and the
disk is 0.50. Determine the linear speed of the coin when it just begins to slip.
If the experiment in part (c) were repeated with a second, identical coin glued to the top of the first coin, how
would this affect the answer to part (c)? Explain your reasoning.
75
AP Physics Multiple Choice Practice – Work-Energy
1.
A mass m attached to a horizontal massless spring with spring constant k, is set
into simple harmonic motion. Its maximum displacement from its equilibrium
position is A. What is the masses speed as it passes through its equilibrium
position?
2.
A force F at an angle Оё above the horizontal is used to pull a heavy suitcase of weight mg a distance d along a
level floor at constant velocity. The coefficient of friction between the floor and the suitcase is Вµ. The work
done by the frictional force is:
(A) –Fd cos θ
(B) – µ Fd cos θ
(C) -Вµmgd
(D) -Вµ mgd cos Оё
3.
A 2 kg ball is attached to a 0.80 m string and whirled in a horizontal circle at a constant speed of 6 m/s. The
work done on the ball during each revolution is:
(A) 90 J
(B) 72 J
(D) 16 J
(D) zero
4.
A pendulum bob of mass m on a cord of length L is pulled sideways until the
cord makes an angle Оё with the vertical as shown in the figure to the right. The
change in potential energy of the bob during the displacement is:
(A) mgL (1–cos θ)
(B) mgL (1–sin θ)
(C) mgL sin Оё
(D) mgL cos Оё
5.
A softball player catches a ball of mass m, which is moving towards her with horizontal speed V. While
bringing the ball to rest, her hand moved back a distance d. Assuming constant deceleration, the horizontal
force exerted on the ball by the hand is
(A) mV2/(2d)
(B) mV2/d
(C) 2mV/d
(D) mV/d
6. A pendulum is pulled to one side and released. It swings freely to the opposite side and stops. Which of the
following might best represent graphs of kinetic energy (E k), potential energy (Ep) and total mechanical energy
(ET)
Questions 7-8: A car of mass m slides across a patch of ice at a speed v with its brakes locked. It the hits dry
pavement and skids to a stop in a distance d. The coefficient of kinetic friction between the tires and the dry
road is Вµ.
7. If the car has a mass of 2m, it would have skidded a distance of
(A) 0.5 d
(B) d
(C) 1.41 d
(D) 2 d
8. If the car has a speed of 2v, it would have skidded a distance of
(A) d
(B) 1.41 d
(C) 2 d
(D) 4 d
9. A ball is thrown vertically upwards with a velocity v and an initial kinetic energy Ek. When half way to the top
of its flight, it has a velocity and kinetic energy respectively of
115
10. A football is kicked off the ground a distance of 50 yards downfield. Neglecting air resistance, which of the
following statements would be INCORRECT when the football reaches the highest point?
(A) all of the balls original kinetic energy has been changed into potential energy
(B) the ball’s horizontal velocity is the same as when it left the kickers foot
(C) the ball will have been in the air one-half of its total flight time
(D) the vertical component of the velocity is equal to zero
11. A mass m is attached to a spring with a spring constant k. If the mass is set into motion by a displacement d
from its equilibrium position, what would be the speed, v, of the mass when it returns to equilibrium position?
(A)
(B)
(C)
(D)
12. A fan blows the air and gives it kinetic energy. An hour after the fan has been turned off, what has happened to
the kinetic energy of the air?
(A) it disappears
(B) it turns into potential energy
(C) it turns into thermal energy
(D) it turns into sound energy
13. A rock is dropped from the top of a tall tower. Half a second later another rock, twice as massive as the first, is
dropped. Ignoring air resistance,
(A) the distance between the rocks increases while both are falling.
(B) the acceleration is greater for the more massive rock.
(C) they strike the ground more than half a second apart.
(D) they strike the ground with the same kinetic energy.
14. Which of the following is true for a system consisting of a mass oscillating on the end of an ideal spring?
(A) The kinetic and potential energies are equal to each other at all times.
(B) The maximum potential energy is achieved when the mass passes through its equilibrium position.
(C) The maximum kinetic energy and maximum potential energy are equal, but occur at different times.
(D) The maximum kinetic energy occurs at maximum displacement of the mass from its equilibrium position
15. From the top of a high cliff, a ball is thrown horizontally with initial speed v o. Which of the following graphs
best represents the ball's kinetic energy K as a function of time t ?
(A)
(B)
(C)
(D)
Questions 16-17: A block oscillates without friction on the end of a spring as
shown. The minimum and maximum lengths of the spring as it oscillates are,
respectively, xmin and xmax. The graphs below can represent quantities
associated with the oscillation as functions of the length x of the spring.
(A)
(B)
(C)
(D)
16. Which graph can represent the total mechanical energy of the block-spring system as a function of x ?
(A) A
(B) B
(C) C
(D) D
116
17. Which graph can represent the kinetic energy of the block as a function of x ?
(A) A
(B) B
(C) C
(D) D
Questions 18-19
A ball swings freely back and forth in an arc from point I to point IV, as
shown. Point II is the lowest point in the path, III is located 0.5 meter above
II, and IV is I meter above II. Air resistance is negligible.
18. If the potential energy is zero at point II, where will the kinetic and
potential energies of the ball be equal?
(A) At point II
(B) At some point between II and III
(C) At point III (D) At some point between III and IV
19. The speed of the ball at point II is most nearly
(A) 3.0 m/s
(B) 4.5 m/s
(C) 9.8 m/s
(D) 14 m/s
20. The figure shows a rough semicircular track whose ends are at a vertical height h. A block placed at point P at
one end of the track is released from rest and slides past the
bottom of the track. Which of the following is true of the height
to which the block rises on the other side of the track?
(A) It is equal to h/4
(B) It is equal to h/2
(C) It is equal to h
(D) It is between zero and h; the exact height depends on how
much energy is lost to friction.
21. The graph shown represents the potential energy U as a function of displacement
x for an object on the end of a spring moving back and forth with amplitude x пЂ°.
Which of the following graphs represents the kinetic energy K of the object
as a function of displacement x ?
22. A child pushes horizontally on a box of mass m which moves with constant speed v across a horizontal floor.
The coefficient of friction between the box and the floor is пЃ­. At what rate does the child do work on the box?
(A) пЃ­mgv
(B) mgv
(C) пЃ­mg/v
(D) пЃ­mg/v
117
AP Physics Free Response Practice – Work Power Energy
1974B1. A pendulum consisting of a small heavy ball of mass m at the end of a
string of length L is released from a horizontal position. When the ball is
at point P, the string forms an angle of Оё with the horizontal as shown.
Оё
(a) In the space below, draw a force diagram showing all of the forces
acting on the ball at P. Identify each force clearly.
(b) Determine the speed of the ball at P.
(c) Determine the tension in the string when the ball is at P.
1974B7. A ski lift carries skiers along a 600 meter slope inclined at 30В°. To lift a single rider, it is necessary to
move 70 kg of mass to the top of the lift. Under maximum load conditions, six riders per minute arrive at the
top. If 60 percent of the energy supplied by the motor goes to overcoming friction, what average power must
the motor supply?
121
1975B1. A 2-kilogram block is released from rest at the top of a curved incline in the shape of a quarter of a circle
of radius R. The block then slides onto a horizontal plane where it finally comes to rest 8 meters from the beginning
of the plane. The curved incline is frictionless, but there is an 8-newton force of friction on the block while it slides
horizontally. Assume g = 10 meters per second2.
a. Determine the magnitude of the acceleration of the block while it slides along the horizontal plane.
b. How much time elapses while the block is sliding horizontally?
c. Calculate the radius of the incline in meters.
1975B7. A pendulum consists of a small object of mass m fastened to the end of an inextensible cord of length L.
Initially, the pendulum is drawn aside through an angle of 60В° with the vertical and held by a horizontal string as
shown in the diagram above. This string is burned so that the pendulum is released to swing to and fro.
a. In the space below draw a force diagram identifying all of the forces acting on the object while it is held by the
string.
b. Determine the tension in the cord before the string is burned.
c. Show that the cord, strong enough to support the object before the string is burned, is also strong enough to
support the object as it passes through the bottom of its swing.
122
1977 B1. A block of mass 4 kilograms, which has an initial speed of 6 meters per second at time t = 0, slides on a
horizontal surface.
a. Calculate the work W that must be done on the block to bring it to rest.
If a constant friction force of magnitude 8 newtons is exerted on the block by the surface, determine the following:
b. The speed v of the block as a function of the time t.
c. The distance x that the block slides as it comes to rest
1978B1. A 0.5 kilogram object rotates freely in a vertical circle at the end of a string of length 2 meters as shown
above. As the object passes through point P at the top of the circular path, the tension in the string is 20 newtons.
Assume g = 10 meters per second squared.
(a) On the following diagram of the object, draw and clearly label all significant forces on the object when it is at
the point P.
(b) Calculate the speed of the object at point P.
(c) Calculate the increase in kinetic energy of the object as it moves from point P to point Q.
(d) Calculate the tension in the string as the object passes through point Q.
123
AP Physics Multiple Choice Practice – Momentum and Impulse
1.
A car of mass m, traveling at speed v, stops in time t when maximum braking force is applied. Assuming the
braking force is independent of mass, what time would be required to stop a car of mass 2m traveling at speed v?
(A) ВЅ t
(B) t
(C) в€љ2 t
(D) 2t
2.
A block of mass M is initially at rest on a frictionless floor. The block, attached to a massless spring with spring
constant k, is initially at its equilibrium position. An arrow with mass m and velocity v is shot into the block.
The arrow sticks in the block. What is the maximum compression of the spring?
( A) v
m
k
( B) v
mпЂ«M
k
(C )
( m пЂ« M )v
mk
( D)
mv
( m пЂ« M )k
3.
Two objects, P and Q, have the same momentum. Q can have more kinetic energy than P if it has:
(A) More mass than P
(B) The same mass as P
(C) More speed than P
(D) The same speed at P
4.
A spring is compressed between two objects with unequal masses, m and M, and held together. The objects are
initially at rest on a horizontal frictionless surface. When released, which of the following is true?
(A) The total final kinetic energy is zero.
(B) The two objects have equal kinetic energy.
(C) The speed of one object is equal to the speed of the other.
(D) The total final momentum of the two objects is zero.
5.
Two football players with mass 75 kg and 100 kg run directly toward each other with speeds of 6 m/s and 8 m/s
respectively. If they grab each other as they collide, the combined speed of the two players just after the
collision would be:
(A) 2 m/s
(B) 3.4 m/s
(C) 4.6 m/s
(D) 7.1 m/s
6. A 5000 kg freight car moving at 4 km/hr collides and couples with an 8000 kg freight car which is initially at
rest. The approximate common final speed of these two cars is
(A) 1 km/h
(B) 1.3 km/h
(C) 1.5 km/h
(D) 2.5 km/h
7. A rubber ball is held motionless a height ho above a hard floor and released. Assuming that the collision with
the floor is elastic, which one of the following graphs best shows the relationship between the total energy E of
the ball and its height h above the surface.
(A)
(B)
(C)
(D)
8. Two carts are held together. Cart 1 is more massive than Cart 2. As they are forced apart by a compressed
spring between them, which of the following will have the same magnitude for both carts.
(A) change of velocity
(B) force
(C) speed
(D) velocity
9. A ball with a mass of 0.50 kg and a speed of 6 m/s collides perpendicularly with a wall and bounces off with a
speed of 4 m/s in the opposite direction. What is the magnitude of the impulse acting on the ball?
(A) 1 Ns (B) 5 Ns (C) 2 m/s (D) 10 m/s
10. A cart with mass 2m has a velocity v before it strikes another cart of mass 3m at rest. The two carts couple and
move off together with a velocity of
(A) v/5
(B) 2v/5
(C) 2v/3
(D) (2/5)1/2 v
176
11. Multiple Correct: Consider two laboratory carts of different masses but identical kinetic energie . Which of the
following statements must be correct? Select two answers.
(A) The one with the greatest mass has the greatest momentum
(B) The same impulse was required to accelerate each cart from rest
(C) Both can do the same amount of work as they come to a stop
(D) The same amount of force was required to accelerate each cart from rest
12. A mass m has speed v. It then collides with a stationary object of mass 2m. If both objects stick together in a
perfectly inelastic collision, what is the final speed of the newly formed object?
(A) v / 3
(B) v / 2
(C) 2v / 3
(D) 3v / 2
13. A 50 kg skater at rest on a frictionless rink throws a 2 kg ball, giving the ball a velocity of 10 m/s. Which
statement describes the skater’s subsequent motion?
(A) 0.4 m/s in the same direction as the ball's motion.
(B) 0.4 m/s in the opposite direction of the ball's motion.
(C) 2 m/s in the same direction as the ball's motion.
(D) 2 m/s in the opposite direction of the ball's motion.
14. A student initially at rest on a frictionless frozen pond throws a 1 kg hammer in one direction. After the throw,
the hammer moves off in one direction while the student moves off in the other direction. Which of the
following correctly describes the above situation?
(A) The hammer will have the momentum with the greater magnitude
(B) The student will have the momentum with the greater magnitude
(C) The hammer will have the greater kinetic energy
(D) The student will have the greater kinetic energy
15. Two toy cars with different masses originally at rest are pushed apart by a spring between them. Which TWO
of the following statements would be true?
(A) both toy cars will acquire equal but opposite momenta
(B) both toy cars will acquire equal kinetic energies
(C) the more massive toy car will acquire the least speed
(D) the smaller toy car will experience an acceleration of the greatest magnitude
16. A tennis ball of mass m rebounds from a racquet with the same speed v as it had
initially as shown. The magnitude of the momentum change of the ball is
(A) 0
(B) 2mv
(C) 2mv sinпЃ±
(D) 2mv cosпЃ±
17. Two bodies of masses 5 and 7 kilograms are initially at rest on a horizontal frictionless surface. A light spring
is compressed between the bodies, which are held together by a thin thread. After the spring is released by
burning through the thread, the 5 kilogram body has a speed of 0.2 m/s. The speed of the 7 kilogram body is
(in m/s)
1
1
1
1
(A) 12
(B) 7
(C) 5
(D)
35
18. Multiple Correct: A satellite of mass M moves in a circular orbit of radius R at a constant speed v around the
Earth which has mass ME. Which of the following statements must be true? Select two answers:
(A) The net force on the satellite is equal to Mv2/2 and is directed toward the center of the orbit.
(B) The net work done on the satellite by gravity in one revolution is zero.
(C) The angular momentum of the satellite is a constant.
(D) The net force on the satellite is equal to GMM E/R
177
19. Two pucks are firmly attached by a stretched spring and are initially held at rest on a frictionless surface, as
shown above. The pucks are then released simultaneously. If puck I has three times the mass of puck II, which
of the following quantities is the same for both pucks as the spring pulls the two pucks toward each other?
(A) Speed
(B) Magnitude of acceleration
(C) Kinetic energy
(D) Magnitude of momentum
20. Which of the following is true when an object of mass m moving on a horizontal frictionless surface hits and
sticks to an object of mass M > m, which is initially at rest on the surface?
(A) The collision is elastic.
(B) The momentum of the objects that are stuck together has a smaller magnitude than the initial momentum of
the less-massive object.
(C) The speed of the objects that are stuck together will be less than the initial speed of the less massive object.
(D) The direction of motion of the objects that are stuck together depends on whether the hit is a head-on
collision.
21. Two objects having the same mass travel toward each other on a flat surface each with a speed of 1.0 meter per
second relative to the surface. The objects collide head-on and are reported to rebound after the collision, each
with a speed of 2.0 meters per second relative to the surface. Which of the following assessments of this report
is most accurate?
(A) Momentum was not conserved therefore the report is false.
(B) If potential energy was released to the objects during the collision the report could be true.
(C) If the objects had different masses the report could be true.
(D) If the surface was inclined the report could be true.
22. A solid metal ball and a hollow plastic ball of the same external radius are released from rest in a large vacuum
chamber. When each has fallen 1 meter, they both have the same
(A) inertia
(B) speed
(C) momentum
(D) change in potential energy
23. A railroad car of mass m is moving at speed v when it collides with a second railroad car of mass M which is at
rest. The two cars lock together instantaneously and move along the track. What is the kinetic energy of the
cars immediately after the collision?
(A) ВЅ mv2
(B) ВЅ (M+m)(mv/M)2
(C) ВЅ (M+m)(Mv/m)2
(D) ВЅ (M+m)(mv/(m+M))2
24. An open cart on a level surface is rolling without frictional loss through a vertical downpour of rain, as shown
above. As the cart rolls, an appreciable amount of rainwater accumulates in the cart. The speed of the cart will
(A) increase because of conservation of mechanical energy
(B) decrease because of conservation of momentum
(C) decrease because of conservation of mechanical energy
(D) remain the same because the raindrops are falling perpendicular to the direction of the cart's motion
178
AP Physics Free Response Practice – Momentum and Impulse
1976B2.
A bullet of mass m and velocity vo is fired toward a block of mass 4m. The block is initially at rest on a
frictionless horizontal surface. The bullet penetrates the block and emerges with a velocity of
vo
3
(a) Determine the final speed of the block.
(b) Determine the loss in kinetic energy of the bullet.
(c) Determine the gain in the kinetic energy of the block.
1978B2. A block of mass M1 travels horizontally with a constant speed vo on a plateau of height H until it comes to
a cliff. A toboggan of mass M 2 is positioned on level ground below the cliff as shown above. The center of the
toboggan is a distance D from the base of the cliff.
(a) Determine D in terms of vo, H, and g so that the block lands in the center of the toboggan.
(b) The block sticks to the toboggan which is free to slide without friction. Determine the resulting velocity of
the block and toboggan.
1981B2. A massless spring is between a 1-kilogram mass and a 3-kilogram mass as shown above, but is not
attached to either mass. Both masses are on a horizontal frictionless table.
In an experiment, the 1-kilogram mass is held in place and the spring is compressed by pushing on the 3-kilogram
mass. The 3-kilogram mass is then released and moves off with a speed of 10 meters per second.
a. Determine the minimum work needed to compress the spring in this experiment.
In a different experiment, the spring is compressed again exactly as above, but this time both masses are released
simultaneously and each mass moves off separately at unknown speeds.
b. Determine the final velocity of each mass relative to the cable after the masses are released.
183
1983B2. A block of mass M is resting on a horizontal, frictionless table and is attached as shown above to a relaxed
spring of spring constant k. A second block of mass 2M and initial speed v o collides with and sticks to the first
block. Develop expressions for the following quantities in terms of M, k, and vo
a. v, the speed of the blocks immediately after impact
b. x, the maximum distance the spring is compressed
184
1984B2. Two objects of masses Ml = 1 kilogram and M2 = 4 kilograms are free to slide on a horizontal frictionless
surface. The objects collide and the magnitudes and directions of the velocities of the two objects before and after
the collision are shown on the diagram above. (sin 37В° = 0.6, cos 37В° = 0.8, tan 37Вє = 0.75)
a. Calculate the x and y components (px and py, respectively) of the momenta of the two objects before and after
the collision, and write your results in the proper places in the following table.
b. Show, using the data that you listed in the table, that linear momentum is conserved in this collision.
c. Calculate the kinetic energy of the two-object system before and after the collision.
d. Is kinetic energy conserved in the collision?
185
1985B1. A 2-kilogram block initially hangs at rest at the end of two 1-meter strings of negligible mass as shown on
the left diagram above. A 0.003-kilogram bullet, moving horizontally with a speed of 1000 meters per second,
strikes the block and becomes embedded in it. After the collision, the bullet/ block combination swings upward, but
does not rotate.
a. Calculate the speed v of the bullet/ block combination just after the collision.
b. Calculate the ratio of the initial kinetic energy of the bullet to the kinetic energy of the bullet/ block combination
immediately after the collision.
c. Calculate the maximum vertical height above the initial rest position reached by the bullet/block combination.
1990B1. A bullet of mass m is moving horizontally with speed vo when it hits a block of mass 100m that is at rest
on a horizontal frictionless table, as shown above. The surface of the table is a height h above the floor. After the
impact, the bullet and the block slide off the table and hit the floor a distance x from the edge of the table. Derive
expressions for the following quantities in terms of m, h, vo, and appropriate constants:
a. the speed of the block as it leaves the table
b. the change in kinetic energy of the bullet-block system during impact
c. the distance x
Suppose that the bullet passes through the block instead of remaining in it.
d. State whether the time required for the block to reach the floor from the edge of the table would now be greater,
less, or the same. Justify your answer.
e. State whether the distance x for the block would now be greater, less, or the same. Justify your answer.
186
AP Physics Multiple Choice Practice – Oscillations
1. A mass m, attached to a horizontal massless spring with spring constant k, is set into simple harmonic motion.
Its maximum displacement from its equilibrium position is A. What is the mass’s speed as it passes through its
equilibrium position?
(A) A
п‚ пЂ (B) A
m
k
(C)
1 k
A m
(D)
1 m
A k
2. A mass m is attached to a spring with a spring constant k. If the mass is set into simple harmonic motion by a
displacement d from its equilibrium position, what would be the speed, v, of the mass when it returns to the
equilibrium position?
md
k
(A) v пЂЅ
п‚ пЂ k
m
(B) v пЂЅ
kd
m
(C) v пЂЅ
kd
mg
(D) v пЂЅ d
k
m
3. Which of the following is true for a system consisting of a mass oscillating on the end of an ideal spring?
(A) The kinetic and potential energies are equal to each other at all times.
(B) The kinetic and potential energies are both constant.
(C) The maximum potential energy is achieved when the mass passes through its equilibrium position.
(D) The maximum kinetic energy and maximum potential energy are equal, but occur at different times.
Questions 4-5: A block oscillates without friction on the end of a spring as shown.
The minimum and maximum lengths of the spring as it oscillates are, respectively,
xmin and xmax. The graphs below can represent quantities associated with the
oscillation as functions of the length x of the spring.
(A)
(B)
(C)
(D)
4.
Which graph can represent the total mechanical energy of the block-spring system as a function of x ?
(A) A
(B) B
(C) C
(D) D
5.
Which graph can represent the kinetic energy of the block as a function of x ?
(A) A
(B) B
(C) C
(D) D
6.
An object swings on the end of a cord as a simple pendulum with period T. Another object oscillates up and
down on the end of a vertical spring also with period T. If the masses of both objects are doubled, what are the
new values for the Periods?
Pendulum
Mass on Spring
T
T 2
(A)
2
(B)
T
T 2
(C) T 2
T
(D) T 2
T
2
288
7. An object is attached to a spring and oscillates with amplitude A
and period T, as represented on the graph. The nature of the
velocity v and acceleration a of the object at time T/4 is best
represented by which of the following?
(A) v > 0, a > 0
(B) v > 0, a < 0
(C) v > 0, a = 0
(D) v = 0, a < 0
8. When an object oscillating in simple harmonic motion is at its maximum displacement from the equilibrium
position. Which of the following is true of the values of its speed and the magnitude of the restoring force?
Speed
Restoring Force
(A) Zero
Maximum
(B) Zero
Zero
(C) Maximum ВЅ maximum
(D) Maximum Zero
9. A particle oscillates up and down in simple harmonic motion.
Its height y as a function of time t is shown in the diagram.
At what time t does the particle achieve its maximum
positive acceleration?
(A) 1 s
(B) 2 s
(C) 3 s
(D) 4 s
10. The graph shown represents the potential energy U as a function of displacement
x for an object on the end of a spring moving back and forth with amplitude xпЂ°.
Which of the following graphs represents the kinetic energy K of the object
as a function of displacement x ?
289
Questions 11-12
A sphere of mass m1, which is attached to a spring, is displaced downward from its equilibrium position as shown
above left and released from rest. A sphere of mass m2, which is suspended from a string of length L, is displaced to
the right as shown above right and released from rest so that it swings as a simple pendulum with small amplitude.
Assume that both spheres undergo simple harmonic motion
11. Which of the following is true for both spheres?
(A) The maximum kinetic energy is attained as the sphere passes through its equilibrium position
(B) The maximum kinetic energy is attained as the sphere reaches its point of release.
(C) The minimum gravitational potential energy is attained as the sphere passes through its equilibrium position.
(D) The maximum gravitational potential energy is attained when the sphere reaches its point of release.
(E) The maximum total energy is attained only as the sphere passes through its equilibrium position.
12. If both spheres have the same period of oscillation, which of the following is an expression for the spring
constant
(A) L / m1g
(B) g / m2L
(C) m2g / L
(D) m1g / L
13. A simple pendulum and a mass hanging on a spring both have a period of 1 s when set into small oscillatory
motion on Earth. They are taken to Planet X, which has the same diameter as Earth but twice the mass. Which of the
following statements is true about the periods of the two objects on Planet X compared to their periods on Earth?
(A) Both are shorter.
(B) Both are the same.
(C) The period of the mass on the spring is shorter; that of the pendulum is the same.
(D) The period of the pendulum is shorter; that of the mass on the spring is the same
Questions 14-15
A 0.l -kilogram block is attached to an initially unstretched spring of force constant k = 40 newtons per meter as
shown above. The block is released from rest at time t = 0.
14. What is the amplitude, in meters, of the resulting simple harmonic motion of the block?
(A)
1
m
40
(B)
1
m
20
(C)
1
m
4
(D)
1
m
2
290
AP Physics Free Response Practice – Oscillations
1975B7. A pendulum consists of a small object of mass m fastened to the end of an inextensible cord of length L.
Initially, the pendulum is drawn aside through an angle of 60В° with the vertical and held by a horizontal string as
shown in the diagram above. This string is burned so that the pendulum is released to swing to and fro.
a. In the space below draw a force diagram identifying all of the forces acting on the object while it is held by the
string.
b. Determine the tension in the cord before the string is burned.
c. Show that the cord, strong enough to support the object before the string is burned, is also strong enough to
support the object as it passes through the bottom of its swing.
d. The motion of the pendulum after the string is burned is periodic. Is it also simple harmonic? Why, or why not?
1983B2. A block of mass M is resting on a horizontal, frictionless table and is attached as shown above to a relaxed
spring of spring constant k. A second block of mass 2M and initial speed v o collides with and sticks to the first
block Develop expressions for the following quantities in terms of M, k, and v o
a. v, the speed of the blocks immediately after impact
b. x, the maximum distance the spring is compressed
c. T, the period of the subsequent simple harmonic motion
293
1995B1. As shown above, a 0.20-kilogram mass is sliding on a horizontal, frictionless air track with a speed of 3.0
meters per second when it instantaneously hits and sticks to a 1.3-kilogram mass initially at rest on the track. The
1.3-kilogram mass is connected to one end of a massless spring, which has a spring
constant of 100 newtons per meter. The other end of the spring is fixed.
a. Determine the following for the 0.20-kilogram mass immediately before the impact.
i. Its linear momentum
ii. Its kinetic energy
b. Determine the following for the combined masses immediately after the impact.
i. The linear momentum
ii. The kinetic energy
After the collision, the two masses undergo simple harmonic motion about their position at impact.
c. Determine the amplitude of the harmonic motion.
d. Determine the period of the harmonic motion.
1996B2. A spring that can be assumed to be ideal hangs from a stand, as shown above.
a. You wish to determine experimentally the spring constant k of the spring.
i. What additional, commonly available equipment would you need?
ii. What measurements would you make?
iii. How would k be determined from these measurements?
b. Assume that the spring constant is determined to be 500 N/m. A 2.0-kg mass is attached to the lower end of the
spring and released from rest. Determine the frequency of oscillation of the mass.
c. Suppose that the spring is now used in a spring scale that is limited to a maximum value of 25 N, but you would like
to weigh an object of mass M that weighs more than 25 N. You must use commonly available equipment and the
spring scale to determine the weight of the object without breaking the scale.
i. Draw a clear diagram that shows one way that the equipment you choose could be used with the spring
scale to determine the weight of the object,
ii. Explain how you would make the determination.
294
2005B2
A simple pendulum consists of a bob of mass 1.8 kg attached to a string of length 2.3 m. The pendulum is held at
an angle of 30В° from the vertical by a light horizontal string attached to a wall, as shown above.
(a) On the figure below, draw a free-body diagram showing and labeling the forces on the bob in the position
shown above.
(b) Calculate the tension in the horizontal string.
(c) The horizontal string is now cut close to the bob, and the pendulum swings down. Calculate the speed of the
bob at its lowest position.
(d) How long will it take the bob to reach the lowest position for the first time?
295